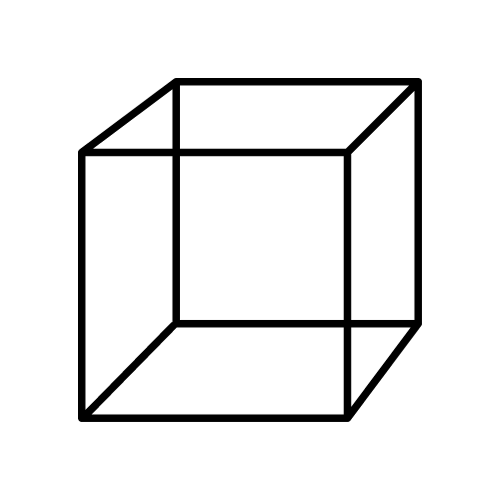
Cube ☰

Volume Formula:

\(V=a^3\)

Surface area of the cube:

\(S=6a^2\)

The area of the lateral surface of the cube:

\(S=4a^2\)

Diagonal:

\(d= a \sqrt{3}\)

The definition:

A rectangular parallelepiped with equal dimensions is called a cube. It has 6 faces, each in the shape of a square, 12 edges, and 8 corner points. The number of edges emanating from a single point is 3.

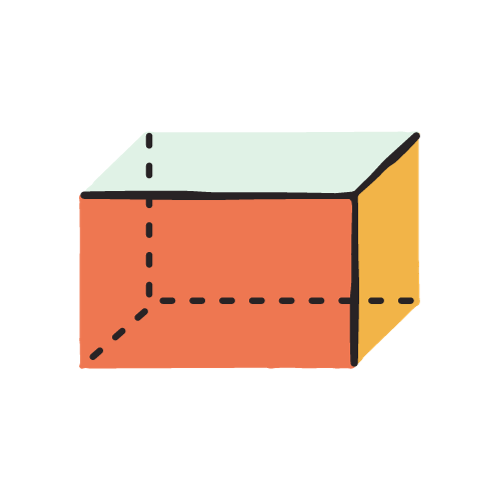
Parallelepiped ☰

Volume formula of a rectangular parallelepiped:

\(V=a \cdot b \cdot c \)

Total surface area:

\( \small S = 2 \cdot ( a \cdot b + a \cdot c + b \cdot c) \)

Lateral Surface area

\(S = 2 \cdot ( a \cdot c + b \cdot c ) \)

Diagonal:

For a Rectangle:

Diagonal (\( \small d\)) = \( \small \sqrt{{\text{Length}^2 + \text{Width}^2}}\)

For a Square:

Diagonal (\( \small d\)) = \( \small \text{Side} \times \sqrt{2}\)

For a Parallelogram:

Diagonal 1 (\( \small d_1\)) = \( \small \text{Side 1} \times \sqrt{2}\)

Diagonal 2 (\( \small d_2\)) = \( \small \text{Side 2} \times \sqrt{2}\)

For a Cube:

Diagonal (\( \small d \)) = \( \small \text{Side} \times \sqrt{3}\)

For a Rectangular Box (Rectangular Parallelepiped):

Diagonal (\( \small d\)) = \( \small \sqrt{{\text{Length}^2 + \text{Width}^2 + \text{Height}^2}}\)

For a Rhombus:

Diagonal 1 (\( \small d_1\)) = \( \small 2 \times \text{Side} \times \sin(\frac{\theta}{2})\), where \(\theta\) is one of the angles.

Diagonal 2 (\( \small d_2\)) = \( \small 2 \times \text{Side} \times \sin(90^\circ - \frac{\theta}{2})\)

The definition:

A parallelepiped is a three-dimensional geometric shape with six parallelogram faces. It is a polyhedron, which means it has flat faces, straight edges, and sharp corners (vertices). The term "parallelepiped" is often used more specifically to refer to a six-faced parallelepiped.

The key characteristics of a parallelepiped are as follows:

1. Six Faces: A parallelepiped has six faces, which are parallelograms. These faces come in pairs, where each face is parallel to another.

2. Edges: It has 12 edges, which are the line segments where two faces meet.

3. Vertices: A parallelepiped has 8 vertices, which are the points where three edges meet.

4. Equal Opposite Faces: The opposite faces are equal in size and shape, and they are parallel to each other.

5. Right Angles: The edges where the faces meet form right angles.

6. Rectangular Base: In the context of a rectangular parallelepiped, its base consists of four right-angled, congruent rectangles.

7. Three Dimensions: The parallelepiped is characterized by its three dimensions: length, width, and height.

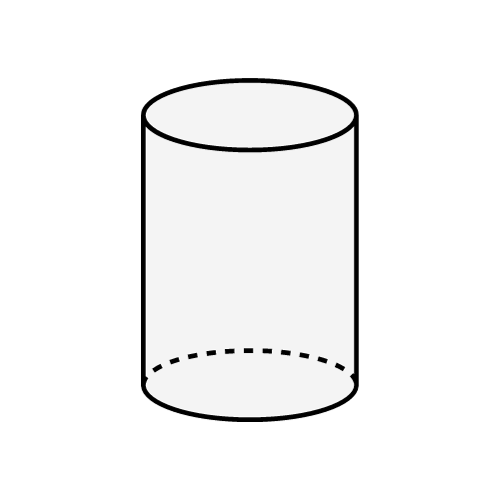
Cylinder ☰

Volume formula:

\(V= \pi r^2 \cdot h\)

Total surface area:

\( \small S = 2 \pi r \cdot h + 2 \cdot \pi r^2 = 2 \pi r(r+h) \)

Lateral surface area:

\(S = 2 \pi r \cdot h \)

The definition:

A cylinder is a three-dimensional geometric shape with two parallel and congruent circular bases and a curved surface connecting these bases. It is one of the most common and fundamental geometric shapes.

Essential characteristics of a cylinder:

Two Circular Bases: A cylinder has two flat, circular ends that are identical in size and shape. These bases are parallel to each other.

Curved Surface: The curved surface of the cylinder connects the two circular bases. It forms a lateral surface that is smooth and does not have any corners or edges.

Axis: The line segment connecting the centers of the two circular bases is called the axis of the cylinder. It is perpendicular to the bases.

Height: The height of the cylinder is the distance between the two circular bases along the axis. It is a measure of how tall the cylinder is.

Radius: The radius is the distance from the center of one of the circular bases to the edge (or perimeter) of that base. The radius is the same for both bases.

Prism ☰

Volume formula:

\(V= S_\text{base} \cdot h \)

Total surface area:

\( \small S = 2 S_\text{base} + S_\text{lateral} \)

Lateral surface area:

\(S = P_\text{base} \cdot l \)

The definition:

A prism is a three-dimensional geometric shape with two parallel and congruent polygonal bases and rectangular or parallelogram faces connecting the corresponding sides of the bases. The essential characteristics of a prism are as follows:

Two Polygonal Bases: A prism has two flat polygonal ends that are identical in size and shape. These bases are parallel to each other.

Rectangular or Parallelogram Faces: The faces of the prism connect the corresponding sides of the bases. These faces are typically rectangular or parallelogram in shape.

Edges: The line segments where the faces meet are called the edges of the prism.

Height: The height of the prism is the perpendicular distance between the two bases. It is a measure of how tall the prism is.

Lateral Faces: The faces that connect the sides of the bases are called the lateral faces. The number of lateral faces is equal to the number of sides in the polygonal bases.

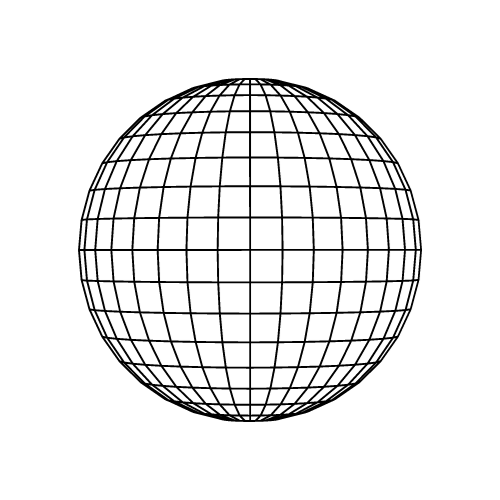
Sphere ☰

Volume formula:

\( V = \frac{4}{3} \pi r^3 \)

Surface area:

\( S = 4 \pi r^2 \)

Diameter:

\( D=2r \)

The definition:

A sphere is a three-dimensional geometric shape that is perfectly round and symmetrical. It is characterized by several essential features:

1. Roundness: A sphere is perfectly round and has no edges or corners. It is defined as the set of all points that are a fixed distance (the radius) away from a central point (the center).

2. Center: The center of the sphere is a point located equidistant from all points on the sphere's surface.

3. Surface: The surface of a sphere is the set of all points that are at the same fixed distance from the center. It forms a continuous, curved surface with no flat sides.

4. Radius: The radius of a sphere is the distance from the center to any point on its surface. All radii of a sphere are of equal length.

5. Diameter: The diameter is a straight line passing through the center of the sphere and connecting two points on the sphere's surface. It is equal to twice the radius.

Spheres are a fundamental geometric shape and are found in various aspects of mathematics, science, and the physical world. They have unique properties, such as having the maximum volume for a given surface area and being highly symmetrical. Spheres are commonly encountered in the study of geometry, astronomy, and engineering.